Grand constructions with Octiamond Ring

In 2017, John Greening, one of the world's outstanding puzzle aficionados based in the UK, custom-ordered our Iamond Ring and Octiamond Ring in special colors and sizes 6, 7, and 8. At the end of July he amazed us with this complex nested-rings solution that he calls the "Grand Iamond Ring", after many arduous efforts at solving. He writes, "What a thrill when the last piece fitted into place!!" Yes, John, we know that thrill, too. Here's John Greening, holding the workboard before completing the solution above. Congratulations, John, on these heroic achievements. Years later, John found related solutions of the nested rings, with shifted positions and different arrangements of the rings:   In 2018 John outdid himself by commissioning us to make him a full set of the 160 enneiamonds: all the shapes of 9 equilateral triangles joined. "This way lies madness," we thought, until John sent us a solution he had found, the fantastic and dramatic figure below. Notice how he managed to get the one piece with a hole to sit right in the center. We wondered what spectacular idea John would come up with next, and here it is. In May 2020, John Greening produced the ultimate (so far) assembly of the hexiamonds through dekiamonds forming concentric rings around a center star, after months of determined hands-on work. No easy computer solutions for this passionate solver. We show his magnificent design, and below it the larger solution boards for a clearer view of the ring arrangements. Notice the symmetrical positions of triangles in the two outer rings that are the pieces with enclosed holes.

Some years before, Jacques Ferroul in France sent us these beautiful variants of the Octiamond Ring surrounding a core of hexiamonds:

In 2021, John Greening surprised us with this shapely figure built of the 66 octiamonds and added this note: "This is my favorite of all the sets and I call it the grandfather set because the set is balanced with even parity, there are no holes and all pieces tile the plane. 12-fold symmetry is also possible with this set."   Congratulations, John. That is a thing of beauty, coaxing all different shapes into exquisite collaboration. And you solve these without a computer, so double wow.   At the end of October 2022, John Greening amazed us once again with this magnificent solution of a giant snowflake. It took him about a week to solve by hand, with a clever shuffle of the last six pieces. What a marvel! And a few months later, his superbly symmetrical Stars arrived. The Figure-8/Infinity double ring greeted us in January 2024. Simply gorgeous! Petals on all edges was another lovely feature.   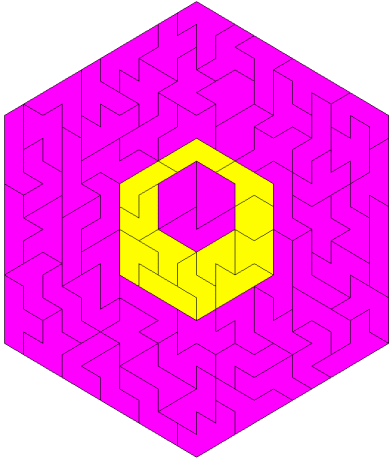On February 3, 2024, John Greening delighted us with this fancy snowflake solution, continuing the six-fold symmetry theme that the octiamonds naturally embrace. Notice the V in the very center. In June 2024, even more elaborate Octiamond designs arrived from John's inventive labors to enrich our gallery: two triple rings, a sausage, and stubby stars. A little later in June 2024, three more beauties arrived. The triangle and the lattice had taken John a few days each to solve. The star took him only about 5 hours.   And as dessert for his summer banquet of successes, John Greening sent exquisite stubby Star formations and a six-fold ball:     In mid-July 2024, John's ingenuity turned to pretty spaces and fancy contours, giving us hexagons with holes, and triangles with triangular holes, and a diamond with a center line of hexagons, and what he called a cloverleaf.And all formed with the tricky tiles of all different shapes of 8 triangles joined. One can only marvel at such solving prowess.     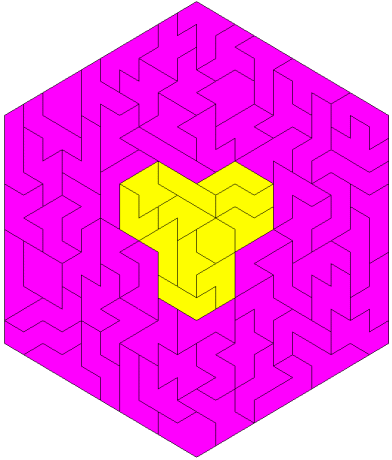Other special features John Greening investigated were symmetrical Octiamond Ring figures that can be formed with either an entire set of the 66 all-different tiles or with 66 copies of only a single shape of tile repeated throughout. Have a look. The single repeating tile shown above each solution could fill the whole pattern.     Then see partnerships of hexiamonds and octiamonds, where the smaller enclosed pieces form precise doubled triamonds or smaller copies of the octiamond figure. See also the "copy-cat" twins. What a delight to know such solutions are even possible!           Here's a pair of handsome Octiamond triangles with three hexiamond hexagons at their center, upside down from each other: — Going one step further, John Greening has combined members of several families into beautiful symmetries—the tetriamonds through heptiamonds embedded into the Octiamond Ring. John's determination is limitless, as the last two figures, the "dogbone" shapes, failed 82 times by a single piece before he turned up these beauties.           Stretched hexagons with various proportions, even with central riders, make another nice group by John Greening: In September 2021, John Greening produced his most amazing result to date: the Hendekiamond Ring, consisting of 1186 different tiles, each 11 triangles in size. At this scale, even more pieces with holes showed up, and John succeeded in arranging all 38 holes (covering 52 unit triangle spaces) in symmetrical locations around the inner and outer perimeter. He even found solutions where the outer triangle holes point either in or out. It took John several months to construct this ring to fit exactly around the previous nested rings (see above), working with painstakingly hand-cut pieces. Rumors came that John was already tackling the next size up—the order-12 polyiamonds with 3,334 different tiles—is that a world record yet?—to be achieved by hand, not by computer. After several weeks, John completed the full ring, with symmetrical arrangements of the pieces with holes. Few humans can equal such a superachievement. To assist in his recording, John built a workboard six feet in diameter, showing the entire solution as it emerged. See the close-up of a smaller section below. See also a close-up of the section around 5 o'clock, where most of the chunky tiles cluster, John's strategy for grouping the easiest pieces for the last. Congratulations, John! You are our greatest winner. We were delighted to receive, on September 18, 2024, a link to a video from John Greening describing his solving process of the 3,334 dodekiamonds, with close-ups of his handcut pieces that cover 26,672 triangles with 6 diamond spaces and 102 single holes, symmetrically distributed in the gigantic hexagon. John's nice voice is amazingly calm, considering the enormously difficult and exciting task he has completed! See and subscribe to his entire YouTube collection

Back to Octiamond Ring